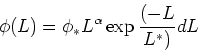

Next: Stellar populations
Up: AY616 class notes
Previous: Observations of galaxies
Subsections
- Numerous physical quantities characterize a galaxy: mass,
stellar/total mass ratio, gas/total mass, size, stellar population,
angular momentum, distribution of orbits, etc.
- These combine to make numerous observational quantities:
luminosity, color, HI flux, morphology, surface brightness,
SB profiles, dynamical properties (e.g.,
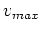 ), etc.
), etc.
- We'd like to know which, if any, of the physical/observable quantities
are correlated with one another to understand the fundamental
driving mechanisms of galaxy formation/evolution.
- Start by considering range of observable properties in galaxies.
- Galaxies population appears to be bimodal
- Might like to consider mass as a possible fundamental parameter,
but mass estimates are difficult; total mass requires dynamical measurements that
are difficult. Stellar mass can be estimated, e.g. by IR luminosity;
estimates can be improved using some estimator of stellar population, e.g.
color and/or spectral features, e.g.
Kauffman et al MNRAS 341, 54 (2003)
- Colors depend on stellar mass, more cleanly than
on luminosity (figure from Baldry et al)
- Stellar populations depend on stellar mass, more cleanly than
on luminosity (figure)
- Structural parameters depend on stellar mass, more cleanly than
on luminosity (figure)
- Appears to be a characteristic stellar mass that divides
galaxy population around a stellar mass of
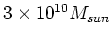 ;
likely this characteristic mass is more related to star formation
processes than to dark matter
;
likely this characteristic mass is more related to star formation
processes than to dark matter
- Gas mass fractions (e.g. Kannappen ApJL 611,89 (2004)
- correlated with color,
- correlated with luminosity
- correlated with stellar mass
- caveat: estimating molecular and hot component of gas more challenging!
- including gas mass with stellar mass provides plausibly better estimator
of underlying mass, as it provides a baryonic mass estimate
- Global properties as a function of morphological type
- Roberts and Haynes review (ARAA 1994):
two samples with optical+HI data,
one from RC3/UGC overlap, another ``volume limited''. Ignore first
since no correction made for selection biases. Remember LSB galaxies are
missing from latter. Masses inferred from HI rotation.
- Find large range in ALL physical quantities for
a given morphological class which is almost certainly NOT attributable to
observational errors or classification errors.
- Considering median or mean
values, find little trend in radius,
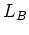 , and total mass for S0-Sc,
but later types tend to be smaller, less massive, and less luminous.
(RH Fig 2).
However, note that LSB galaxies tend to be later types, and, since
these are not included, there may be a bias here.
, and total mass for S0-Sc,
but later types tend to be smaller, less massive, and less luminous.
(RH Fig 2).
However, note that LSB galaxies tend to be later types, and, since
these are not included, there may be a bias here.
- Typical SB may be
lower for latest types, even without inclusion of LSB galaxies; CSB much
higher for large E's than S's. Mass surface density appears to decrease
monotonically with morphological class in spirals.
(RH Fig 3).
- Cold gas absent in
ellipticals, and HI content appears to increase monotonically with type
for spirals. Possible that molecular gas content decreases with type,
but uncertain.
(RH Fig 4).
- Structurally, there is no obvious correlation with morphological type
for the disks:
McGaugh, Schombert and Bothun Fig 1 (5/95). There is an
obvious correlation for B/D, but note there is large range of B/D within
any given morphological class. Obvious difference between E's and S's.
- One possibility is that the range in physical parameters is small among
early-type spirals, and large along late type spirals which have larger
fraction of LSBs, but morphological classification emphasizes distinctions
which can only be seen at HSB!
- Spectroscopically: color decreases monotonically with spectral type
(RH Fig 5).
This can be extended to spectra features as well; the
Hubble sequence is well-correlated with integrated spectral ``types''.
(see Kennicutt 1992, Kinney et al figures for UV behavior).
It appears that this is NOT caused by decreasing B/D alone (see
Kennicutt et al, ApJ 435, 22, 1994).
Note that both E's and S's have color and line-strength
gradients; these are caused by combinations of dust (in spirals)
and true variations in metallicity and/or age, such that inner regions
are redder and probably more metal-rich. Spectroscopic evidence relates
to stellar populations, however.
- Standard suggestion is that later types have more current star
formation, as suggested by spectroscopy and HI content. However,
within spirals, possible problems from sample selection and from
bulge contamination. Possible alternative is that the Hubble sequence
represents different ratio of current star formation to previous
star formation, i.e., not depending on absolute value of current
SFR.
- Still, we haven't identified any fundamental property which indicates
why stellar pops differ among different types. Fact that there's a wide
range of all properties within any type suggests that morphology is
not a fundamental variable, at least within spirals. Fairly clear that
there is a fundamental difference between ellipticals and spirals.
- Environment: galaxies come in wide range of environments, from isolated
galaxies to groups to poor clusters to rich clusters. All generally result
from large scale structure of galaxy distribution.
- Dressler morphology-density relation: clusters contain predominantly
ellipticals, ``field'' contains predominantly spirals
- Postman Geller (ApJ 281, 95, 1984)
extension to groups:
morphology-density relation is a function of local
density.
- Environmental dependence of color sequences
(Baldry et al, MNRAS 373. 469 (2006),
Patiri, Prada, Holtzman, Klypin, Betancourt-Rijo, MNRAS 372, 1710 (2006) )
- Relative number of galaxies in red and blue sequences is a strong function
of environment
- Location of sequences is not a strong function of environment
- luminosity functions give relative numbers of galaxies as
a function of luminosity, both overall and as a function of
morphological type. This may provide additional clues about formation.
- luminosity function of galaxies is an important
cosmological probe for evolution of the galaxy population, as we'll
discuss more later.
- knowledge of the LF is also essential to correct
for systematic biases in mag/diameter limited samples as discussed
previously.
- observed luminosity, however, depends on stellar population;
ideally, one might want to correct for stellar population effects and
derive mass functions, but this requires more information (e.g. spectra),
perhaps even better get dynamical mass functions, but this requires
even more information!
- Galaxy LF
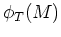 gives number of galaxies per unit volume of
type T in
absolute mag M to M+dM. Summed over type is called general or universal
LF. However, since we've seen that morphological type fraction depends on
environment, we know that general function will depend on environment if
the LF for each type is different.
gives number of galaxies per unit volume of
type T in
absolute mag M to M+dM. Summed over type is called general or universal
LF. However, since we've seen that morphological type fraction depends on
environment, we know that general function will depend on environment if
the LF for each type is different.
- Note distinction between luminosity distribution, which just gives relative
numbers of galaxies at different luminosities, and luminosity function,
which also gives number of galaxies per volume element. The latter is
a product of luminosity distribution with density function. This nomenclature
isn't commonly accepted, and for now we'll just call either thing the
luminosity function.
- measuring the LF
- relatively simple in a cluster (beware of foreground/background).
- In the field, need to know selection function.
- Also, need to beware since galaxies are not uniformly distributed
in space, so just using V/Vmax can lead to significant errors.
Binggelli et al (ARAA 26, 26, 1988)
discusses techniques, and is a good general summary.
- LFs usually parametrized by a Schecter function:
where 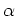 is the faint-end slope.
is the faint-end slope.
- Useful feature of Schecter function is that it can be integrated to get
total luminosity density:
- Results:
- Schematic cluster and field LFs.
- Different types have clearly different LFs.
- Since proportion of a given type depends on environment, so must general
LF. So far, no strong evidence that LF of a given type depends on environment.
- Note distinction between E's and dE's.
- Note cD's don't fit.
- Note Schecter fit is only an approximation; typical
parameters are
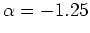 for clusters,
for clusters, 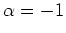 for field,
for field,
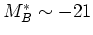 ; dependence on type (e.g.
Marzke Fig 3)
; dependence on type (e.g.
Marzke Fig 3)
- Can easily see evidence for morphology-density relation.
E , S0, dE strong suppressed in the field; later spiral types
more enhanced than earlier ones in the field as well.
- Some more recent results:
- LSB galaxies and their effect on the LF;
- Driver-Cross overview figure
the
- luminosity-SB relation
of galaxies and it's possible effect on the
determination of luminosity functions.
- Possible that different selection a source of the variations observed
in LF from survey to survey, e.g. SB or Clustering/morphology as a
possibility for variations in faint end slope.
- Large scale structure as a possibility for variations in normalization
of luminosity function.
- Galaxy stellar mass function
(e.g. Panter, Heavans, & Jimenez, NMRAS 355, 764, 2004
- Note characteristic shape of luminosity or stellar mass function
differs from that of predicted dark matter halo masses
(e.g. Benson et al., ApJ 599, 38, 2003


Next: Stellar populations
Up: AY616 class notes
Previous: Observations of galaxies
Jon Holtzman
2007-05-04
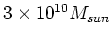 ;
likely this characteristic mass is more related to star formation
processes than to dark matter
;
likely this characteristic mass is more related to star formation
processes than to dark matter
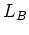 , and total mass for S0-Sc,
but later types tend to be smaller, less massive, and less luminous.
(RH Fig 2).
However, note that LSB galaxies tend to be later types, and, since
these are not included, there may be a bias here.
, and total mass for S0-Sc,
but later types tend to be smaller, less massive, and less luminous.
(RH Fig 2).
However, note that LSB galaxies tend to be later types, and, since
these are not included, there may be a bias here.